Dr. Autar Kaw receives a grant to lead improvement of flipped classrooms through adaptive learning
Tampa, FL (December 20, 2016) – Ever since Autar Kaw started teaching at the University of South Florida in 1987, he has used evidence-based pedagogies such as interactive instructional software and active learning in the classroom. Since 2001, he has been conducting federally funded research in transforming undergraduate engineering education through development and assessment of open resources for undergraduate courses such as Numerical Methods.
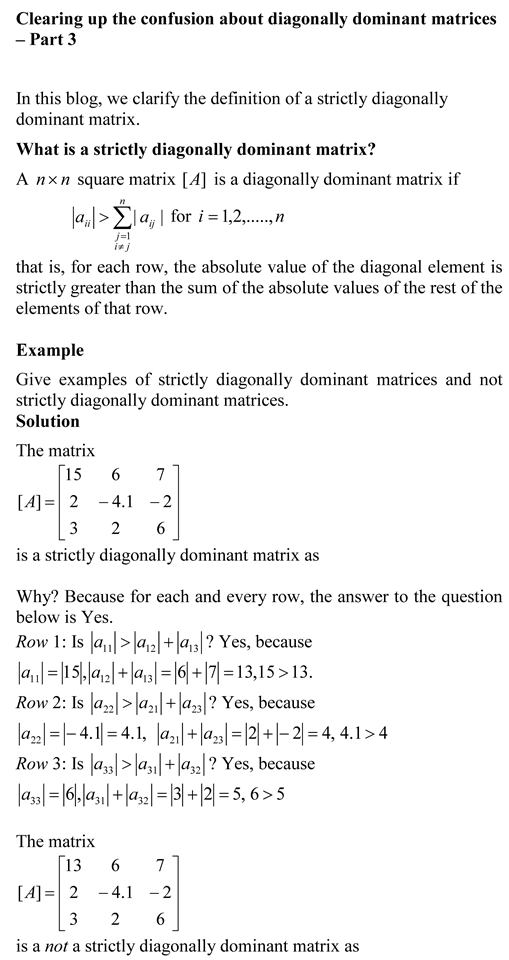
With his success of using blended learning for more than a decade and having been the lead developer of a comprehensive open courseware for Numerical Methods, the National Science Foundation (NSF) granted a four-institution (University of South Florida, Arizona State University, Alabama A&M, and University of Pittsburgh) grant in 2013 to determine the effectiveness of flipped learning classroom. As per the Flipped Learning Network, “Flipped Learning is a pedagogical approach in which direct instruction moves from the group learning space to the individual learning space, and the resulting group space is transformed into a dynamic, interactive learning environment where the educator guides students as they apply concepts and engage creatively in the subject matter.” The research from the grant indicated positive improvement in student learning gains through flipped learning but challenges persisted in some students coming inadequately prepared for the classroom meeting. Although before class they were required to study through their preference of video lectures or textbook, and take an automatically-graded online quiz, it still was a single recipe prescribed for all.
To address this concern of pre-class preparation, NSF has given Professor Kaw and his colleague Professor Mary Besterfield-Sacre of University of Pittsburgh an Engaged Student Learning Exploration and Design grant that will use the adaptive platform of Knewton, Inc to personalize the pre-class activities for every learner. Knewton’s mission is to support and challenge every student to meet their learning goals through personalized learning.
Traditional vs Flipped Classroom (Photo Courtesy of University of Washington – Center for Teaching and Learning and Office of the Provost)
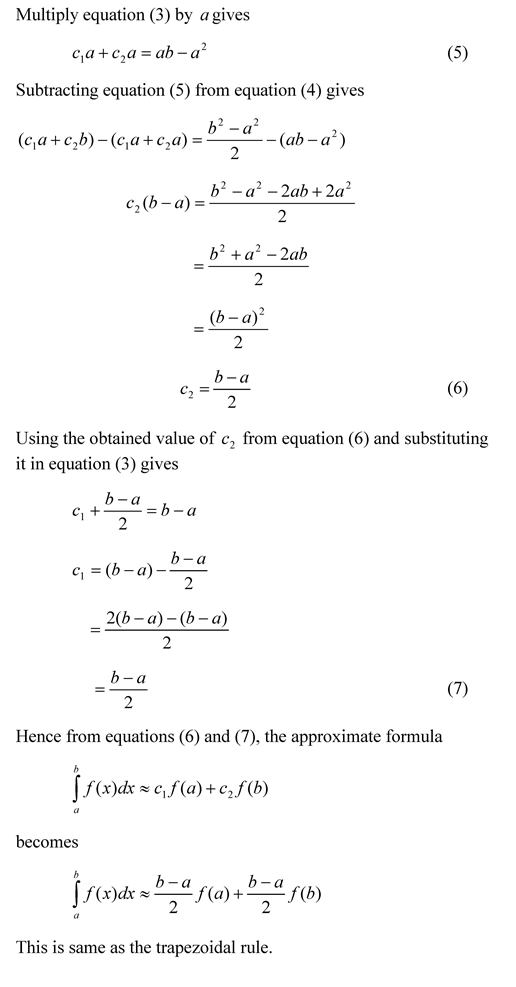
To improve the pre-class activities of the flipped class, the instructional and assessment modules from prior NSF support are being augmented and revised to conform to the Knewton adaptive platform. Specifically, the project will be comparing the effectiveness of three instructional approaches: a flipped class with adaptive learning, a flipped class without adaptive learning, and a blended class without adaptive learning, based on student conceptual gains, procedural knowledge, higher-order problem solving, and affective learning. These comparisons will be conducted statistically at a granular level using the factors of GPA, gender, race, age, transfer status, socioeconomic status, working hours, course topic, proficiency levels, and time on task, as well as qualitatively via focus groups and interviews. This work will hence advance the understanding of the impact of the combined flipped and adaptive approaches on cognitive and affective learning gains of students representing diverse populations.
This project will specifically provide materials and best practices for teaching Numerical Methods in a flipped setting on an adaptive platform and provide new viable opportunities to how STEM courses are taught. This effort will also take us closer to meeting the NAE’s 21st Century Grand Challenge of “Advance Personalized Learning”. Dissemination avenues include the freely-available Knewton adaptive platform, an open education resource portal from prior support, and social media.
For more information about the project, send an email to kaw@usf.edu.
