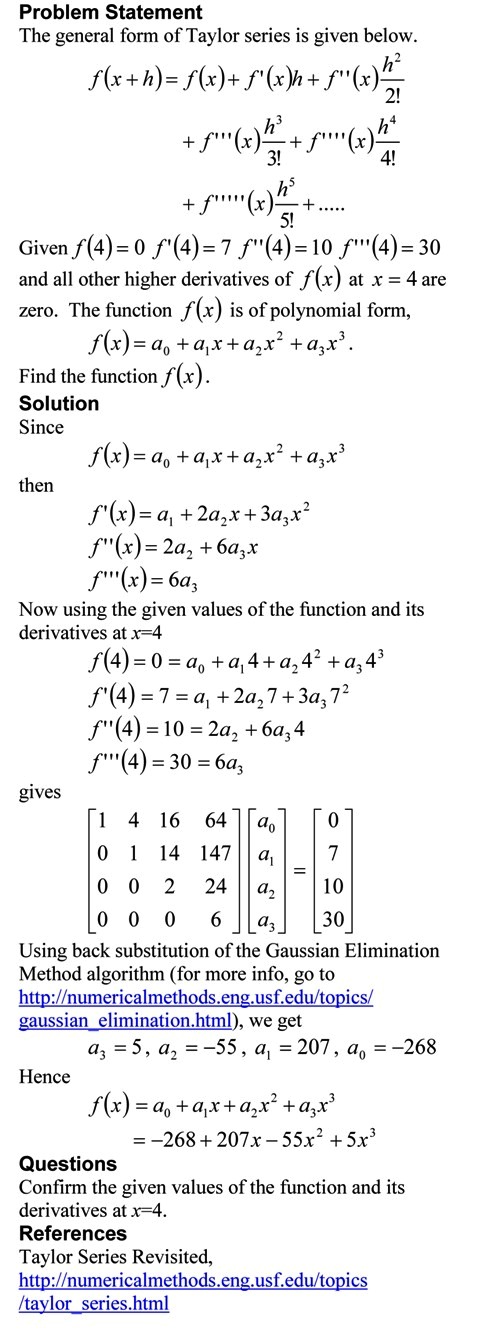
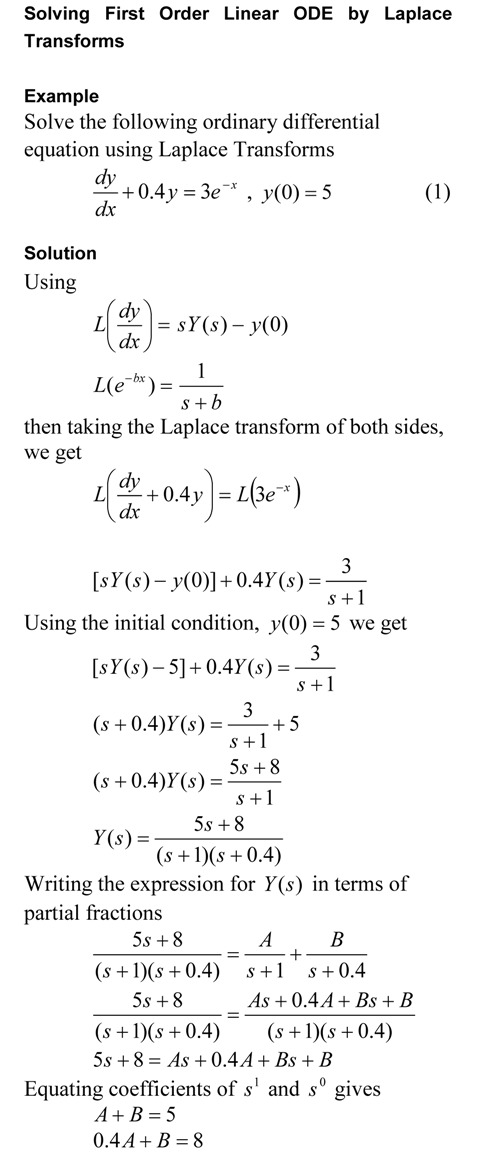
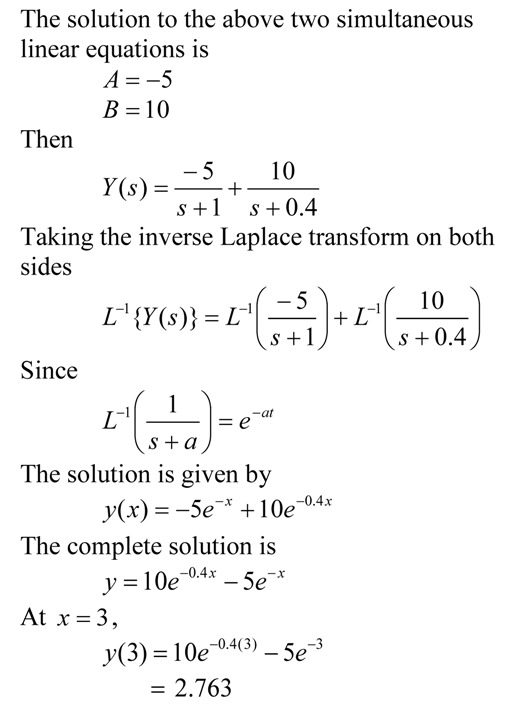
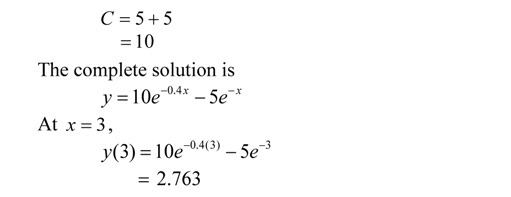The Taylor series for a function f(x) of one variable x is given by
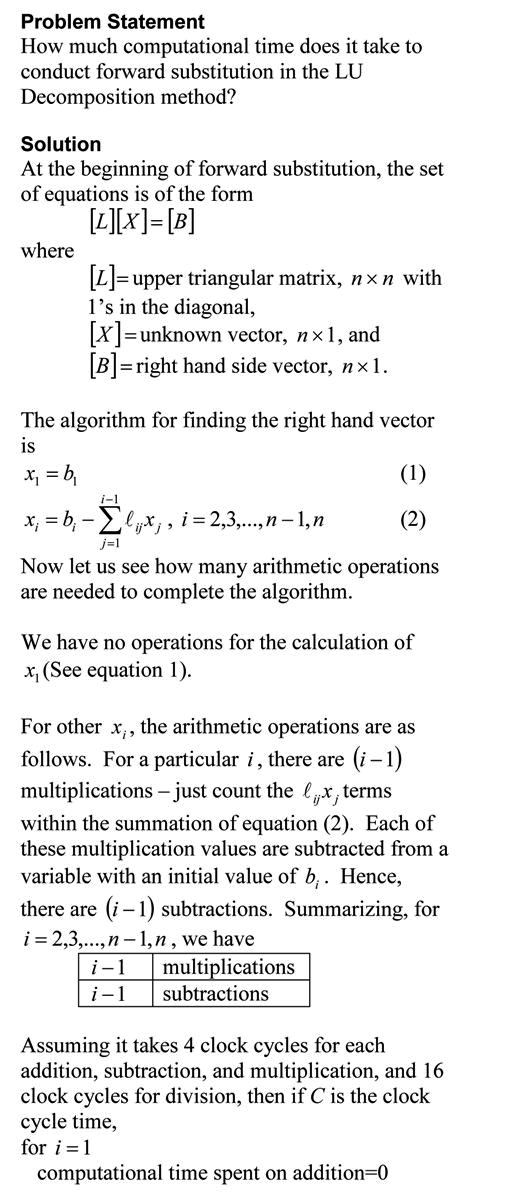
f(x+h) = f(x) + f '(x)h +f ''(x) h^2/2!+ f ''' (x)h^3/3! + .............
What does this mean in plain English?
As Archimedes would have said (without the fine print), “Give me the value of the function at a single point, and the value of all (first, second, and so on) its derivatives, and I can give you the value of the function at any other point”.
It is very important to note that the Taylor series is not asking for the expression of the function and its derivatives, just the value of the function and its derivatives at a single point.
Now the fine print: Yes, all the derivatives have to exist and be continuous between x (the point where you are) to the point, x+h where you are wanting to calculate the function at. However, if you want to calculate the function approximately by using the n^{th} order Taylor polynomial, then 1^{st}, 2^{nd} ……., n^{th} derivatives need to exist and be continuous in the closed interval [x, x+h] , while the n+1^{th} derivative needs to exist and be continuous in the open interval (x, x+h).
Reference: Taylor Series Revisited
This post is brought to you by
Subscribe to the blog via a reader or email to stay updated with this blog. Let the information follow you.