I have a audiovisual digital lecture on YouTube that shows the use of Euler’s method to solve a first order ordinary differential equation (ODE). To show the accuracy of Euler’s method, I compare the approximate answer to the exact answer. A YouTube viewer asked me: How did I get the exact answer?
In this blog, I use the Laplace transform technique to find the exact answer to the ODE. In a previous blog, I showed how to find the exact answer to the ODE by the classicial solution technique.
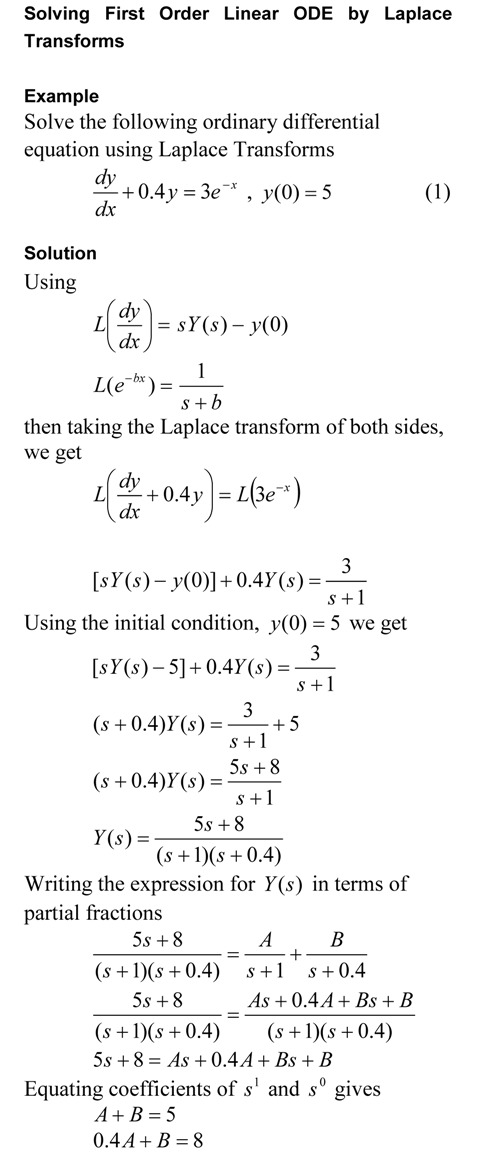
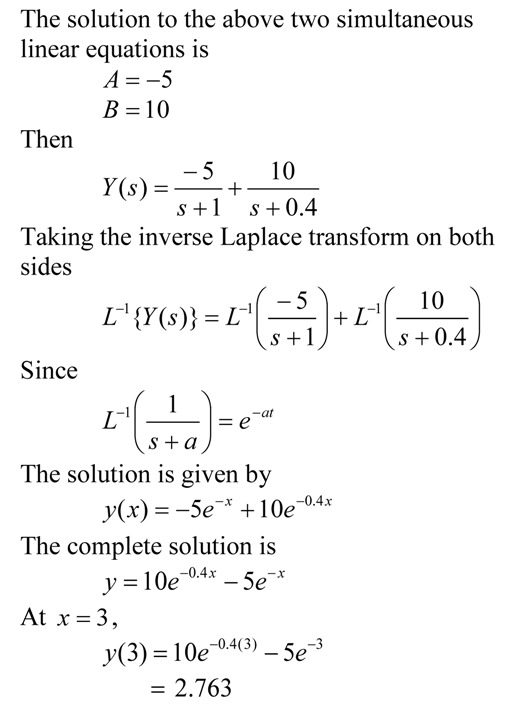
The pdf file of the solution is also available.
indeed the above relations can be applied to Lagrangian expression as say prof dr mircea orasanu and prof horia orasanu
indeed the above relations can be applied to Lagrangian expression as say prof dr mircea orasanu and prof horia orasanu
in more and many circumstances we meet important aspects as look prof dr mircea orasanu as observed follow with
LAGRANGIAN AND FOURIER ANALYSIS
ABSTRACT
Thus an explicit closed-form control for an uncertain, nonlinear, nonautonomous multi-body system that satisfies trajectory control requirements placed on the nominal system (to within pre-specified error bounds), is obtained. An example of a triple pendulum in which there are uncertainties both in the description of its physical parameters and in the description of the gravity field in which it moves is considered. The example demonstrates the simplicity and efficacy of the approach when there are uncertainties both in the description of a dynamical system and in the given forces to which it is subjected.
in more and many circumstances we meet important aspects as look prof dr mircea orasanu as observed follow with
LAGRANGIAN AND FOURIER ANALYSIS
ABSTRACT
Thus an explicit closed-form control for an uncertain, nonlinear, nonautonomous multi-body system that satisfies trajectory control requirements placed on the nominal system (to within pre-specified error bounds), is obtained. An example of a triple pendulum in which there are uncertainties both in the description of its physical parameters and in the description of the gravity field in which it moves is considered. The example demonstrates the simplicity and efficacy of the approach when there are uncertainties both in the description of a dynamical system and in the given forces to which it is subjected.
with the above considerations are admitted important aspects in these context and applied the Laplace transform of LAPLACE equation for harmonic functions and complex functions used by prof drd horia orasanu for followed studies of CONSTRAINTS OPTIMIZATIONS that is coordinate by prof dr Constantin Udriste
with the above considerations are admitted important aspects in these context and applied the Laplace transform of LAPLACE equation for harmonic functions and complex functions used by prof drd horia orasanu for followed studies of CONSTRAINTS OPTIMIZATIONS that is coordinate by prof dr Constantin Udriste